Balancing Act

Weight

Gravity is able to exert a torque on objects too, causing the object to spin (and often fall over). The point where the weight of an object acts on it is called the center of mass (or c.o.m.) The torque from the weight on this bar would cause the bar to swing counterclockwise around the pivot. In the example on the right, the weight exerts a counterclockwise torque on the bar, which will cause the bar to fall (i.e. rotate counterclockwise). For a symmetrical object, the center of mass will usually lie along the axes of symmetry so long as the mass is evenly distributed.

Normal Force

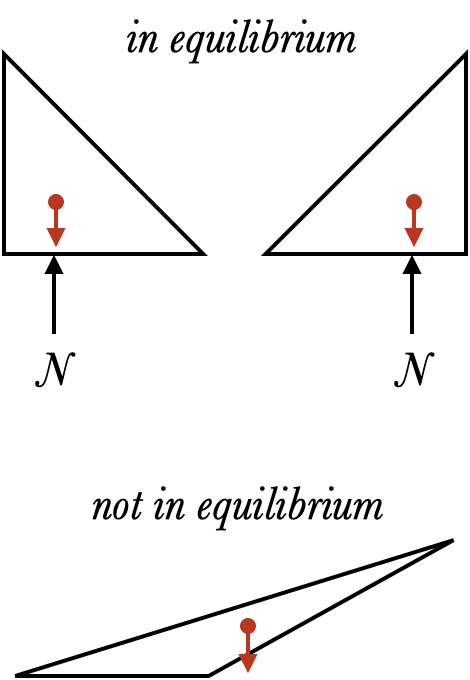
In a lot of examples we've seen, the normal force acts over an area of contact, not at a single point. And just as the normal force will adjust its value to balance other forces, the normal force at a surface can also adjust its location. You may be familiar with this phenomenon when you're standing and you "shift your weight" to the front or back of your foot, even when your entire foot remains flat on the ground. The normal force shifts its position in order to maintain equilibrium, when it can. For an object resting on the floor or the table, this means that the normal force will position itself to be directly under the center of mass.

The normal force can only move within the confines of the surface of contact, however. If the base of the object isn't underneath the center of mass, then the object will tip, as shown on the right.

This demo will let you play around with the torques associated with weight and the normal force on a block standing on the floor. You might explore the following phenomena:

• See if you can balance the block on its corner. Where does the center of mass have to be for that to happen?
• See why a block with a lower center of mass tends to be more stable (that is, is less likely to topple over).
• See why a wider block is more stable than a skinnier block.