Action at a Distance

Imagine that charges are like people: with billions of people on Earth, how do we keep track of each other? One way is through direct communication: we text our friends, or we call or email them, to let them know how we're doing. The other way is through broadcast communication: we set up a Facebook or Twitter account or a blog, we report there how we're doing, and then our friends can catch up on the news at their leisure. In terms of communication, the second way is more efficient (though more lonely perhaps), and this is how charges communicate with one another (as they don't get lonely). Specifically, each charge broadcasts their existence to the universe, and other charges react to that broadcast by feeling a force.

To be more specific: every charge creates an electric field around itself, distorting all of space. A positive charge distorts space so that other positive charges will have a tendency to move away from it, while negative charges have a tendence to move towards it. Other charges in the vicinity cause their own distortions, and the sum total of those distortions determines how other charges will move through that space. The moving charges don't have to know anything about the individual charges that created the distortion; they only need to know what the distortion is like.

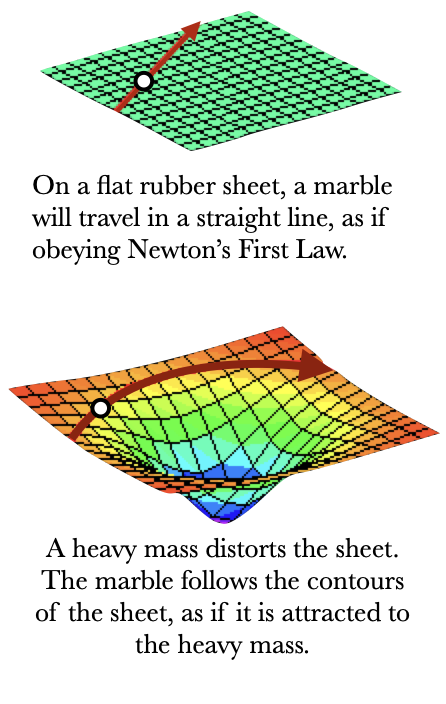
A classic example of how this works is the rubber sheet model, although it is usually used to describe gravity. Here is a good video of the process: imagine that space is typically a flat rubber sheet. If you roll a marble across the sheet, it will travel in a straight line, as Newton's First Law would suggest. However, if you put a heavy mass in the middle of the sheet, it causes a dip in the rubber sheet. Now when I roll the marble across the sheet, it will bend towards the heavy mass, not because the two are attracted to one another, but because the marble is reacting to the distortion in the sheet.

It's important to note that while we show the marble reacting to the rubber sheet, it is also distorting the rubber sheet itself, and other marbles in the vicinity would react to that distortion. Trying to figure out the forces on every object in a scenario could quickly become untenable, so in most cases we will usually single out one object of interest, called the target, and study the forces on that object due to the other objects, which we will call sources. This is very similar to what we did in mechanics when studying forces. In a problem where a block slides down a ramp which sits on a table, for instance, we were usually concerned about the forces on the block, not the forces on the ramp or the table or the ground or anything else in the problem. The block was the target, while the other objects (those that exerted forces directly on the block, at least) were the sources. In the context of fields, we can then say that