Vector Basis and Notation

Adding vectors graphically can be fun, but it would be frustrating to get precise answers out of it. Sometimes we need numbers.

Before we can write a vector this way we must specify a basis: that is, we need to define a positive x-direction, and a positive y-direction perpendicular to it. (If we're working in 3D we will need a "z" as well.) The conventional basis is the one shown here: with $x$ pointing to the right and $y$ pointing up, but you should not assume that this is always the case.

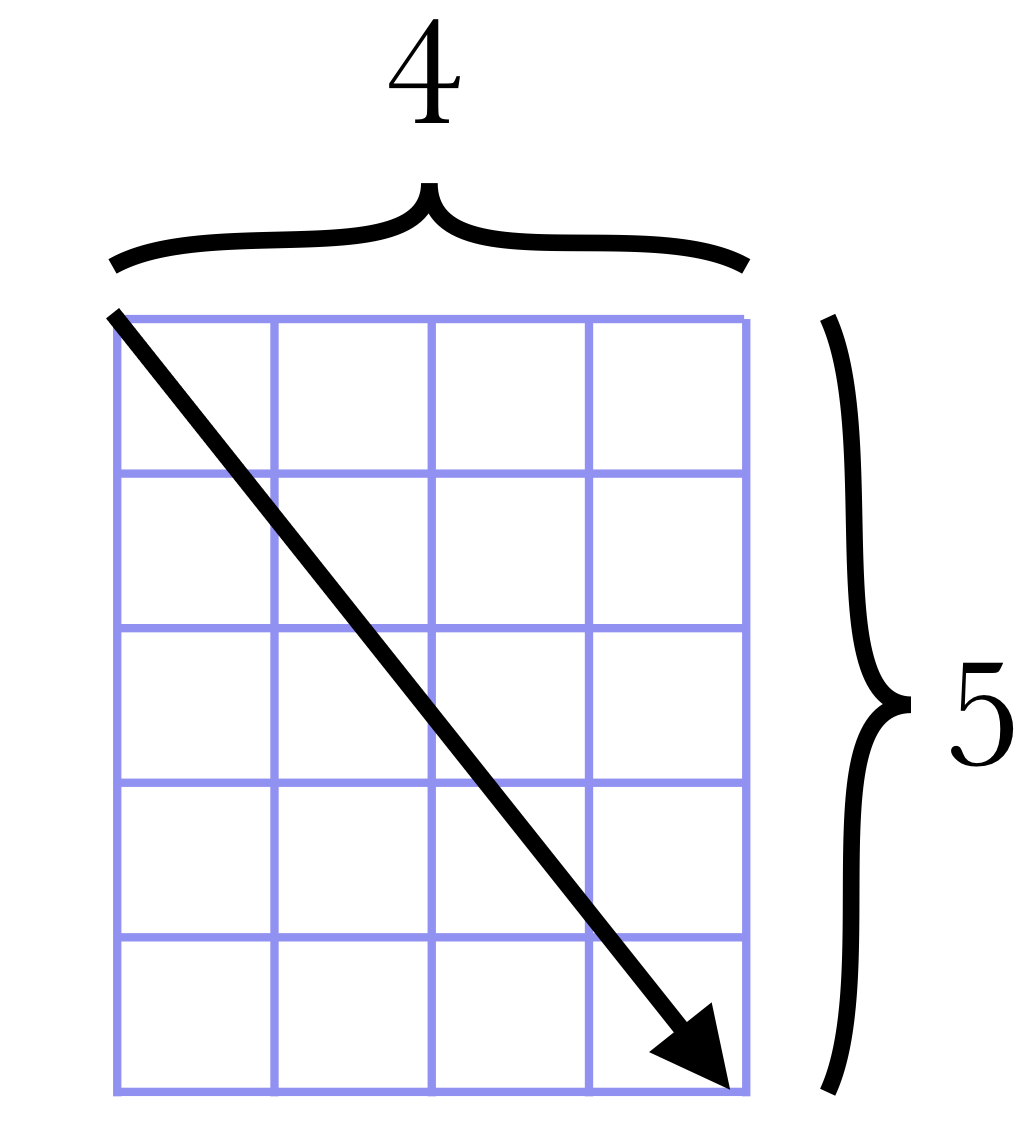
We can write vectors as a set of two or three numbers, called a tuple, like this force: $\vec{F} = (4, - 5) N$ . The individual numbers 4 and –5 are called components of the vector, and are written $F_{x} = 4$ and $F_{y} = - 5$ . The picture on the right shows the vector (4,–5) given the conventional basis above: the vector goes “over 4 and down 5”.

There is nothing particularly special about the conventional basis; we can use whatever basis we want.. For instance, we could make x point down and y point to the right. In that case, the same vector would be written (5,4).

Demo

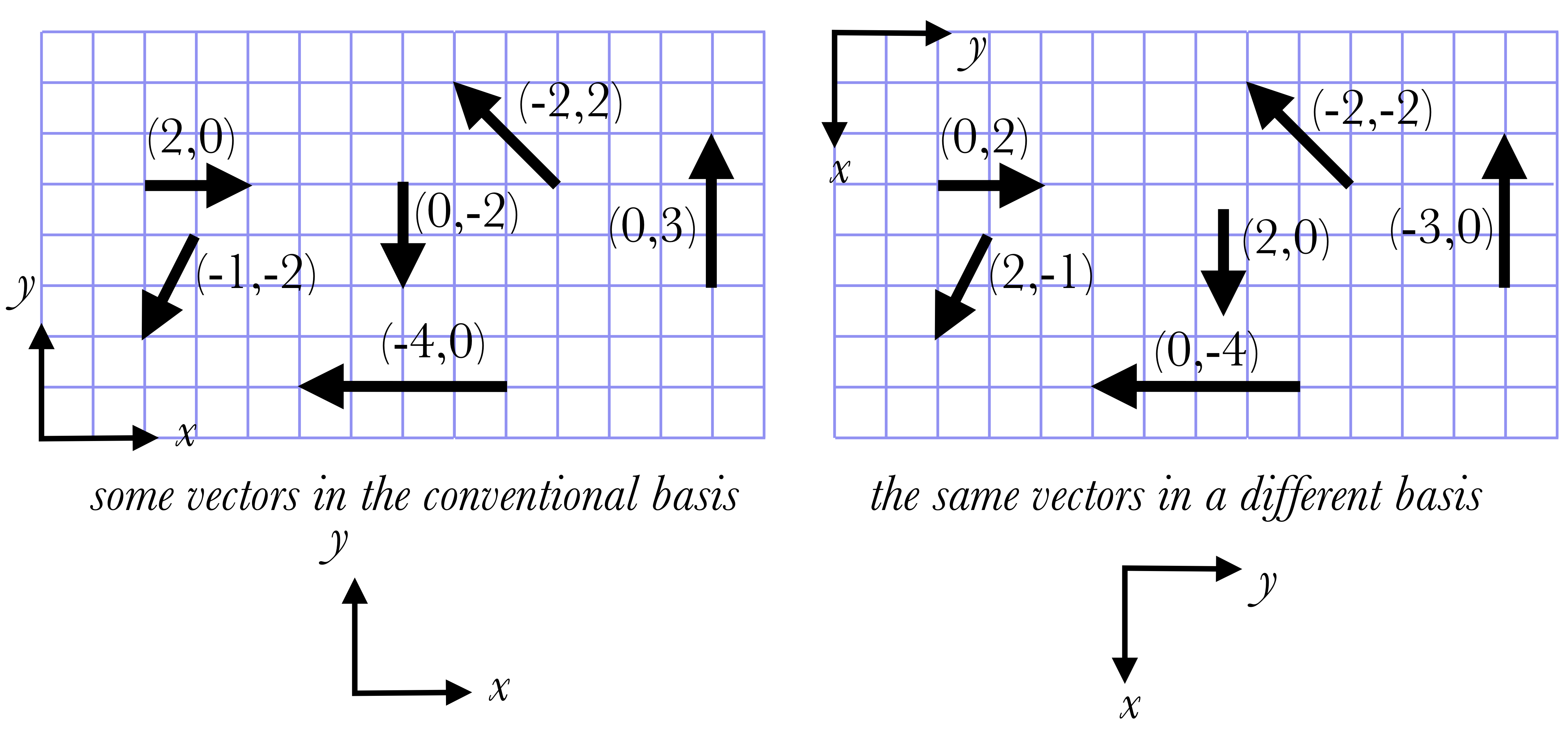
Footnote

Instead of using tuple notation, physicists will often write vectors using unit-vector notation, which looks like this:

\vec{F} = (F_{x}, F_{y}, F_{z}) = F_{x} \hat{i} + F_{y} \hat{j} + F_{z} \hat{k} or F_{x} \hat{x} + F_{y} \hat{y} + F_{z} \hat{z}

So for example, they would write

(3, - 5)

3 \hat{i} - 5 \hat{j}

3 \hat{x} - 5 \hat{y}

The symbols with the hats, like $\hat{x}$ , are called unit vectors. The unit vectors $\hat{i}$ and $\hat{x}$ are vectors that point in the positive- $x$ direction, which have a length of 1. (Not 1 meter or 1m/s or anything, just "1".) Thus $3 \hat{x}$ is a vector which also points in the positive $x$ direction, but which has a length of $3$ .

We won't use this notation in this book, but you may see it elsewhere.