\(\def \u#1{\,\mathrm{#1}}\) \(\def \abs#1{\left|#1\right|}\) \(\def \ast{*}\) \(\def \deg{^{\circ}}\) \(\def \tau{\uptau}\) \(\def \ten#1{\times 10^{#1}}\) \(\def \redcancel#1{{\color{red}\cancel{#1}}}\) \(\def \BLUE#1{{\color{blue} #1}}\) \(\def \RED#1{{\color{red} #1}}\) \(\def \PURPLE#1{{\color{purple} #1}}\) \(\def \th#1,#2{#1,\!#2}\) \(\def \lshift#1#2{\underset{\Leftarrow\atop{#2}}#1}}\) \(\def \rshift#1#2{\underset{\Rightarrow\atop{#2}}#1}}\) \(\def \dotspot{{\color{lightgray}{\circ}}}\) \(\def \ccw{\circlearrowleft}\) \(\def \cw{\circlearrowright}\)
Chapter 4: Rotational Motion
2.

Angular Velocity

Angular velocity is a measure of how fast an object is spinning. It is written with the Greek lowercase letter "omega" $\omega$. Just as velocity is the displacement an object has moved divided by the time it takes, the angular velocity is the angular displacement divided by the time it took:
$$\omega = {\Delta\theta\over\Delta t}$$

We typically measure angular velocity in units of radians per second, although you could also talk about "degrees per second", "revolutions per second", or "revolutions per minute" (RPM, which is commonly used to describe engines.)

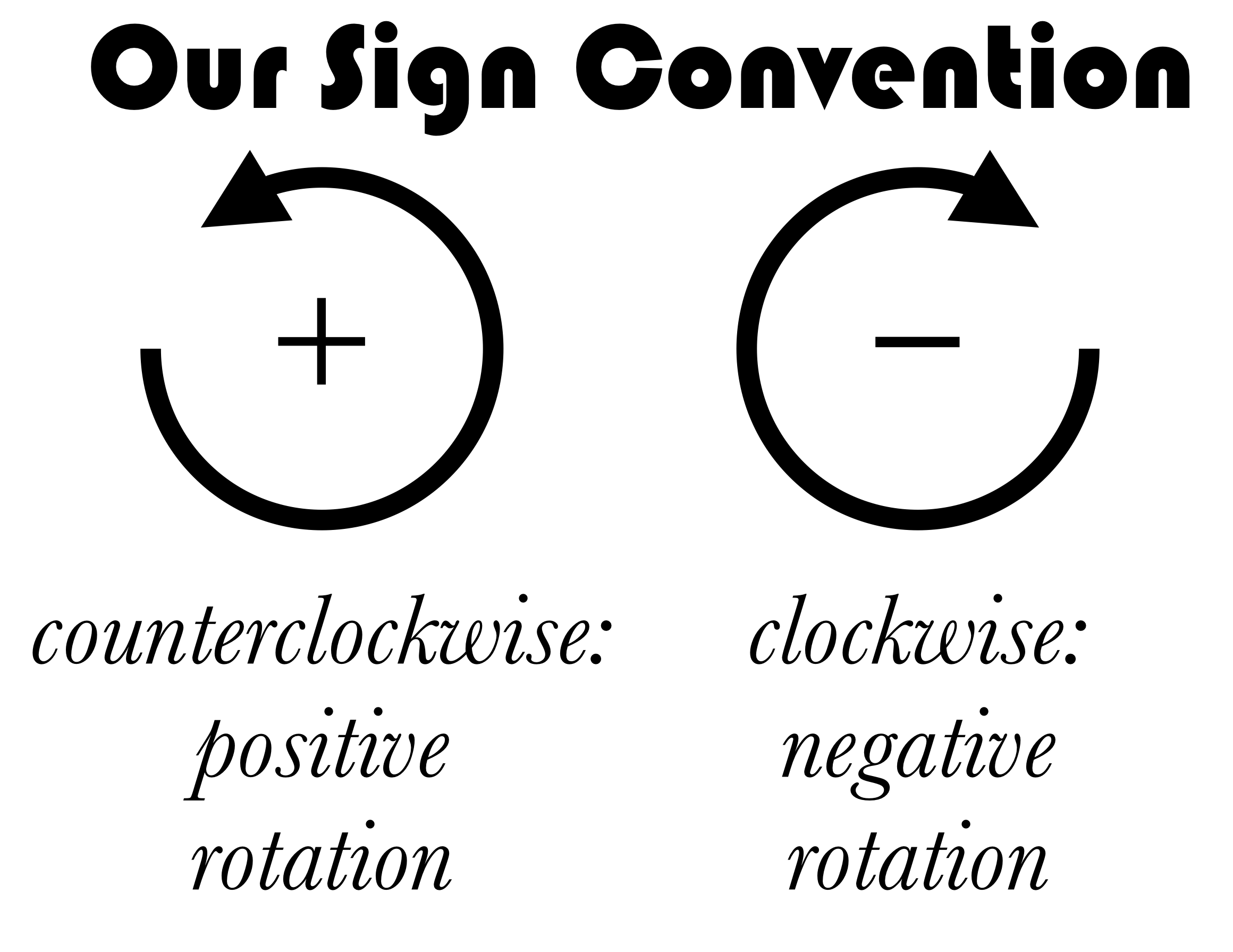
Because an object can spin in one of two opposite directions around an axis, it is useful to define one of these directions as positive, and one as negative. Defining the positive rotational direction is the same as defining the positive x-axis: the choice is completely arbitrary, and so long as you are consistent your results will be in the same. Just to simplify things, in this text we will use the convention that a counterclockwise spin is positive and a clockwise spin is negative. Again this is completely arbitrary; in fact, if you spin your finger clockwise when facing another person, they will see it as counterclockwise motion.