\(\def \u#1{\,\mathrm{#1}}\) \(\def \abs#1{\left|#1\right|}\) \(\def \ast{*}\) \(\def \deg{^{\circ}}\) \(\def \tau{\uptau}\) \(\def \ten#1{\times 10^{#1}}\) \(\def \redcancel#1{{\color{red}\cancel{#1}}}\) \(\def \BLUE#1{{\color{blue} #1}}\) \(\def \RED#1{{\color{red} #1}}\) \(\def \PURPLE#1{{\color{purple} #1}}\) \(\def \th#1,#2{#1,\!#2}\) \(\def \lshift#1#2{\underset{\Leftarrow\atop{#2}}#1}}\) \(\def \rshift#1#2{\underset{\Rightarrow\atop{#2}}#1}}\) \(\def \dotspot{{\color{lightgray}{\circ}}}\) \(\def \ccw{\circlearrowleft}\) \(\def \cw{\circlearrowright}\)
Chapter 3: Linear Motion
8.

Frames of Reference

When you're in a car driving down a straight highway at constant speed, things inside seem pretty normal: you can take a drink, you can throw a ball in the air, you can drop a book, and physics works the same way no matter how fast the car is moving. We call this Galilean relativity. The car in this case is what we call a frame of reference: anyone in the car or moving along with the car sees things working in the same way.

To someone in a different frame of reference, such as outside standing by the roadside, the behavior of the objects in the car will look different. For instance, if I drop a ball straight down inside the car, to someone outside it looks like the ball is travelling through an arc, as is seen in this motion diagram:

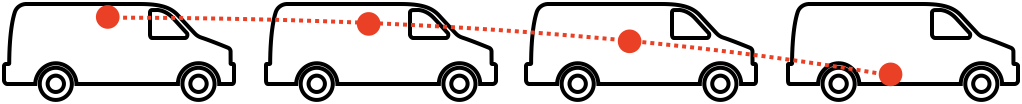
However, while a person in the car and a person on the ground may disagree on what the ball actually does, they both see the ball obeying the same laws of physics. The arc that the ball travels in the above picture is the same arc that it would follow if it was shot horizontally from a cannon with the speed of the car.

We say that a frame of reference is inertial if it is moving at a constant velocity. If the frame is accelerating, such as in a car that is speeding up or going around a curve, we say that it is non-inertial. In a non-inertial frame of reference, physics appears to work a little differently. For example, when a car speeds up, you feel pressed into the seat by a force. If the car suddenly stops, you are thrown forward. There are these apparent extra forces, called pseudoforces, which do not exist in an inertial frame.

While a passenger inside the car would swear that this force exists, an observer in an inertial frame would disagree:

Column

Inertial View

(no alternate text)

The person is speeding up and so the net force must be equal to $m\vec a$, and points to the right. The seat is what supplies that force and so the passenger feels the back of the seat press against them. $$\vec N=m\vec a$$

Column

Non-Inertial View

(no alternate text)

Inside the car's frame, the passenger isn't accelerating at all: they are stationary, so \(F_{net}=0\). However, they can feel the seat pushing them forward; therefore there must be some other "force" balancing the normal force, a pseudoforce equal to $-m\vec a$: $$\vec N+(-m\vec a)=0$$

The equation is the same in both pictures: the difference is which side the acceleration is on. This phenomenon makes your body a pretty good detector of acceleration, by the way. If you're moving and feel pushed in one direction with no forces to account for it, it suggest that you may be accelerating in the opposite direction.