Thin Spherical Devices
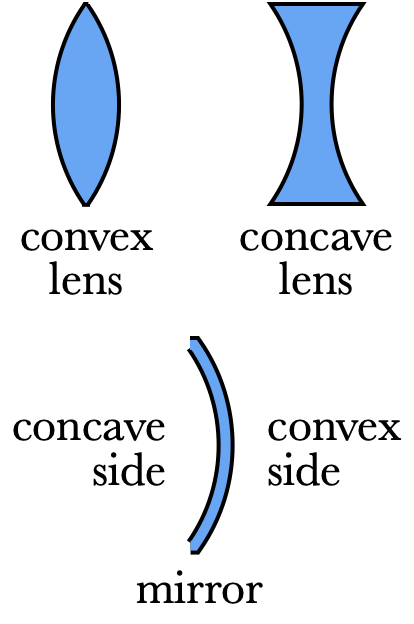
Two types of devices which are commonly used to create images are lenses (shaped glass) and curved mirrors. These are often described by the shape of their surfaces: concave means that the surface bends inward (like a "cave"), while convex means that the surface bends outward (it "flexes" outward?) In this book, however, we will more often describe them in terms of their function:
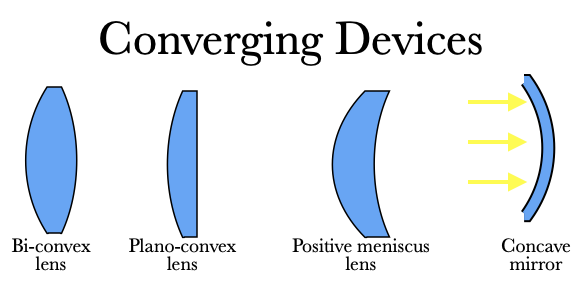
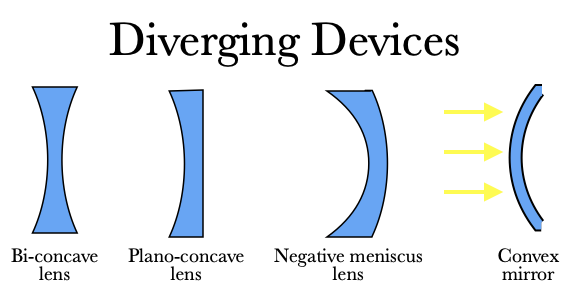
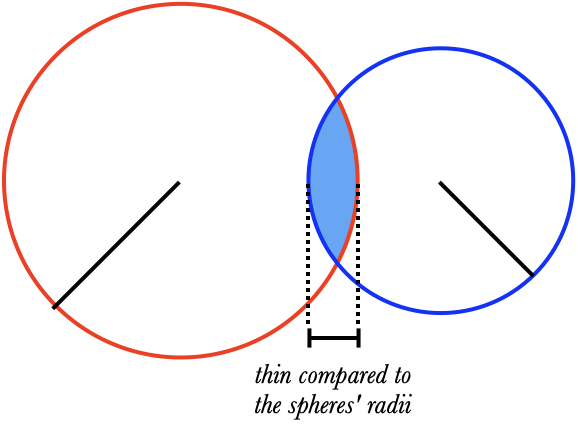
We will especially focus on thin spherical devices, because they are mathematically simpler to work with. A thin spherical device is one whose surfaces are segments of spheres, and where the width of the device is much smaller than the radii of those spheres (hence "thin"). When we talk about lenses and mirrors in the rest of this book, we will always mean thin spherical ones.