How Pressure Varies with Depth

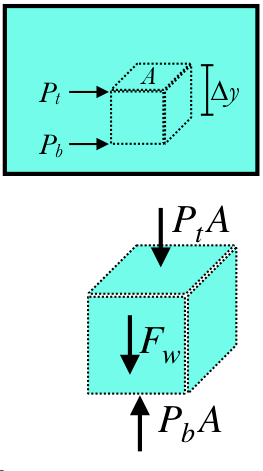
As an object descends into a body of fluid, the pressure that the fluid exerts on the object increases. To see why, consider a cubic region of fluid with height $\Delta y$, whose top and bottom surfaces have area $A$. Fluid exerts pressure on everything it touches, including other bits of fluid, and so the cube feels force on it from the fluid that surrounds it. If the fluid pressure at the top of the cube is $P_t$, then the cube feels a force $F_t=P_tA\downarrow$ on the top surface. The bottom of the cube feels a pressure $P_b$ and an upward force of $F_b=P_bA\uparrow$. The cube experiences a third force, due to the weight of the fluid: $F_w = mg$. The volume of the cube is $V = A\Delta y$ and its mass is $m = \rho_f V = \rho_f A\Delta y$ (where $\rho_f$ is the density of the fluid); therefore the weight of the cube is $F_w = \rho gA\Delta y \downarrow$. These forces must balance each other, so $$\rho gA\Delta y+P_tA=P_bA \implies P_b-P_t=\rho g\Delta y$$ In other words, the change in pressure between any two points in a fluid depends on how deep one point is as compared to the other:

That lets us calculate changes in pressure, but how can we calculate the actual pressure? To do that, we need to know what the pressure $P_0$ is at some reference point. Then the pressure at some point a distance $d$ below the reference point is

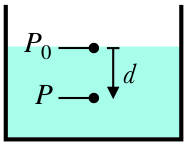
For example, if the fluid is in contact with a gas such as the atmosphere, then the pressure at the surface of the fluid is the same as that of the gas: if the pressure in the fluid were larger, than the fluid would rise; if smaller, it would fall. Such a surface is often a useful reference point: the pressure of the open atmosphere, for instance, is typically $P_{\mathrm{atm}} = 1.01 \times 10^5 \u{Pa}$, as mentioned earlier.