\(\def \u#1{\,\mathrm{#1}}\) \(\def \abs#1{\left|#1\right|}\) \(\def \ast{*}\) \(\def \deg{^{\circ}}\) \(\def \tau{\uptau}\) \(\def \ten#1{\times 10^{#1}}\) \(\def \redcancel#1{{\color{red}\cancel{#1}}}\) \(\def \BLUE#1{{\color{blue} #1}}\) \(\def \RED#1{{\color{red} #1}}\) \(\def \PURPLE#1{{\color{purple} #1}}\) \(\def \th#1,#2{#1,\!#2}\) \(\def \lshift#1#2{\underset{\Leftarrow\atop{#2}}#1}}\) \(\def \rshift#1#2{\underset{\Rightarrow\atop{#2}}#1}}\) \(\def \dotspot{{\color{lightgray}{\circ}}}\) \(\def \ccw{\circlearrowleft}\) \(\def \cw{\circlearrowright}\)
Chapter 6: Energy
8.

Height from What?

There is a certain ambiguity in the gravitational potential energy expression $E_G=mgh$: where should we measure the height from?

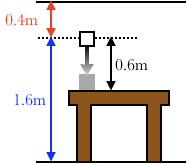
The box starts 0.6m above the table, and then lands on the table.
For example, consider this 5kg box which falls onto this 1-meter tall table. If we measure the height from the table top, then $E_G=29\u{J}$. Or we might measure the height from the floor, giving us $E_G=78\mathrm{J}$. Or someone might even decide to measure the height from the ceiling, giving us $E_G=-20\u{J}$. Which one is correct?
Measuring from... ...the table ...the floor ...the ceiling
  $0.6\u{m} \text{ to } 0\u{m}$ $\BLUE{1.6\u{m}\text{ to } 1\u{m}}$ $\RED{-0.4\u{m}\text{ to }-1.0\u{m}}$
Initial $E_G$ $=(5\u{kg})(9.8\u{N/kg}) \mathbf{(0.6m)}$$29\u{J}$ $=(5\u{kg})(9.8\u{N/kg}) \mathbf{\BLUE{(1.6m)}}$$78\u{J}$ $=(5\u{kg})(9.8\u{N/kg}) \mathbf{\RED{(-0.4m)}}$$-20\u{J}$
Final $E_G$ $=(5\u{kg})(9.8\u{N/kg}) \mathbf{(0m)}$0\u{J}$ $=(5\u{kg})(9.8\u{N/kg}) \mathbf{\BLUE{(1m)}}$ $49\u{J}$ $=(5\u{kg})(9.8\u{N/kg}) \mathbf{\RED{(-1m)}}$$-49\u{J}$
Change in $E_G$ $0\u{J}-29\u{J}$$-29\u{J}$ $49\u{J}-78\u{J}$ $-29\u{J}$ $-49\u{J}-(-20\u{J})$$-29\u{J}$

In fact all of them could be correct. The actual gravitational potential energy of the box is physically irrelevant; it is only changes in potential energy that are important, because those changes are what cause work to be done on objects.

You can measure the height from any reference point you choose,

and so long as you are consistent throughout the problem, the physical behavior of the system will remain the same. You might compare this with the choice of the positive x and y directions when solving a kinematics problem: another person might make a different choice, but you will both describe the same physical phenomenon. One consequence of this fact is that the fact that an object has "zero" gravitational potential energy has no significance whatsoever: that only means that the object is currently located at the height you've chosen as your reference height.