Math with Vector Notation

To multiply or divide a vector by a scalar in this tuple notation, you distribute the scalar over the tuple, multiplying or dividing both components: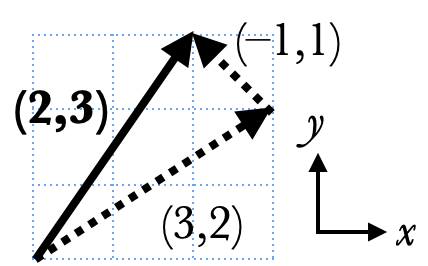

3 (1, 2) = (3, 6) (3, 6) \div 3 = (1, 2)

To add two vectors together in this notation, you add the $x$ values and the $y$ values separately: $(3, 2) + (- 1, 1) = (3 - 1, 2 + 1) = (2, 3)$

The magnitude of a vector $(x, y)$ is $| (x, y) | = \sqrt{x^{2} + y^{2}}$ For example, the magnitude of the vector (2,3) above is $| (2, 3) | = \sqrt{2^{2} + 3^{2}} = \sqrt{4 + 9} = \sqrt{13} \approx 3.6$