Angular Acceleration

Angular acceleration is a measure of how the angular velocity of a spinning object is changing:

α = \frac{Δ ω}{Δ t}

While there are many possible units to measure angular acceleration in, the SI units $rad / s^{2}$ are by far the most common.

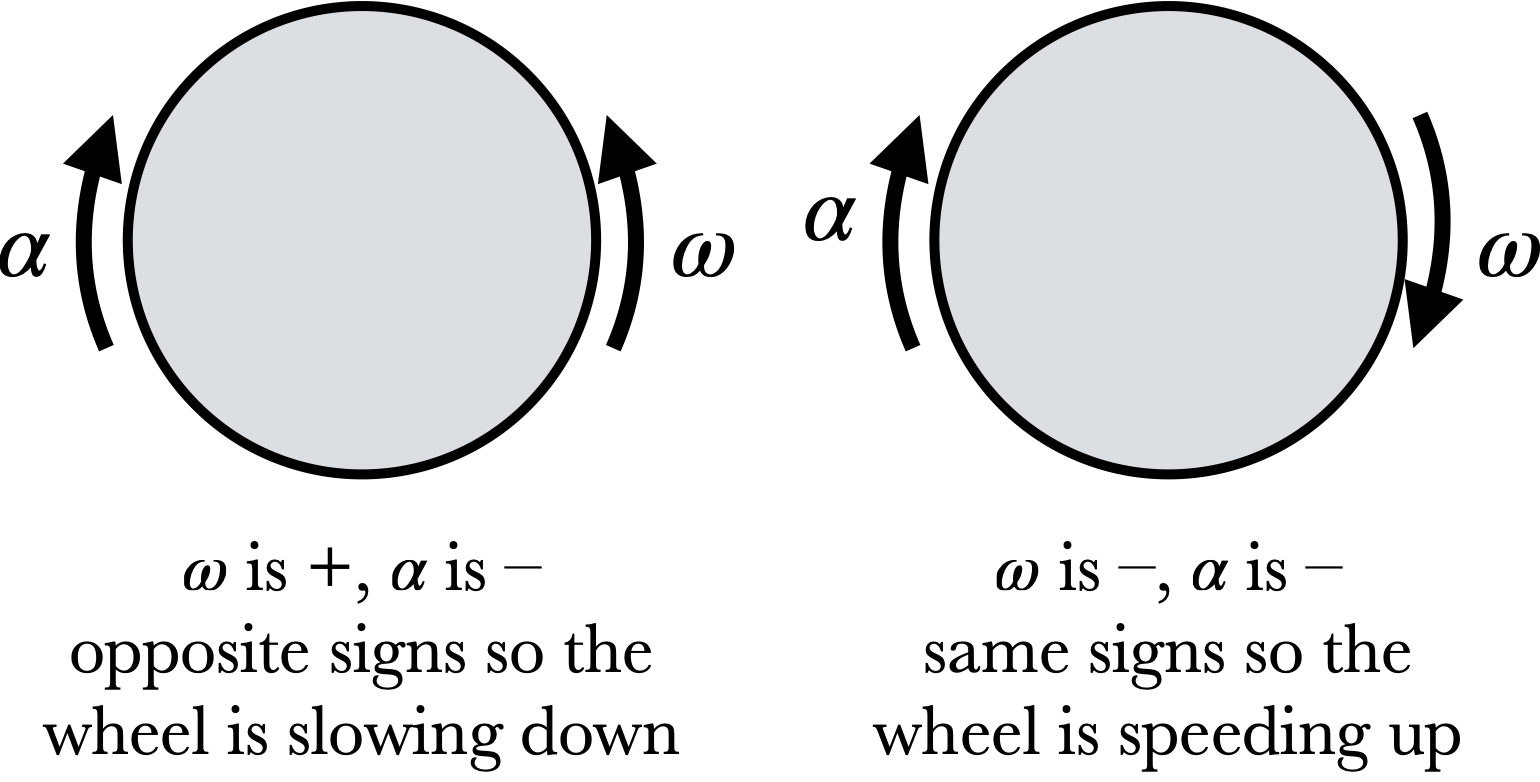
Angular acceleration can point in different directions the way angular velocity can, and the same sign conventions hold. Just as with linear acceleration, an object spins faster if its angular velocity and angular acceleration have the same sign, and an object's spin slows down if they have opposite signs.

Just as linear acceleration comes from an imbalance of forces, angular acceleration comes from an imbalance of torques, as we saw in the section [Missing Link]. $α = \frac{τ_{n e t}}{I}$

Example

Consider a wheel spinning clockwise around an axle, so that its angular velocity is negative. The wheel experiences kinetic friction with the axle, which points in the opposite direction of motion, so the net torque is counterclockwise: thus the angular acceleration is also counterclockwise— that is, positive. Because

α

and

ω

have opposite signs, that means that the wheel is slowing down to the friction.