\(\def \u#1{\,\mathrm{#1}}\) \(\def \abs#1{\left|#1\right|}\) \(\def \ast{*}\) \(\def \deg{^{\circ}}\) \(\def \tau{\uptau}\) \(\def \ten#1{\times 10^{#1}}\) \(\def \redcancel#1{{\color{red}\cancel{#1}}}\) \(\def \BLUE#1{{\color{blue} #1}}\) \(\def \RED#1{{\color{red} #1}}\) \(\def \PURPLE#1{{\color{purple} #1}}\) \(\def \th#1,#2{#1,\!#2}\) \(\def \lshift#1#2{\underset{\Leftarrow\atop{#2}}#1}}\) \(\def \rshift#1#2{\underset{\Rightarrow\atop{#2}}#1}}\) \(\def \dotspot{{\color{lightgray}{\circ}}}\) \(\def \ccw{\circlearrowleft}\) \(\def \cw{\circlearrowright}\)
Chapter 3: Linear Motion
4.

Choosing the Time Interval

We say that $\bar v=\Delta x/\Delta t$ is the average velocity, because the motion diagram can't give us an exact picture of what the car is doing. For instance, the following is a valid motion diagram of a rollercoaster car.

This motion diagram doesn't really capture the behavior of the car. In this case, we should have used a much smaller time interval so that the dots are closer together.

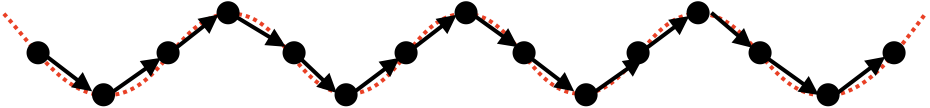
But it is still valid to say that the average velocity between each pair of dots points to the right, even if the car isn't actually moving in that direction.

For a more pragmatic example, suppose someone wants to drive from Toledo to Chicago. We would say that they need to drive west, but really they have to start by driving south first to get to the turnpike. Their speed and direction will vary many times during the course of their trip, but if it takes 4 hours to make the 240 mile trip, then their average velocity is 60mi/hr to the west.